P129. Work on an Inclined Plane

This a combined lab and problem exercise. You'll need some results from L122, and you'll take some initial data. Then you'll carry out calculations using the data and the concept of work.

Aids for your work:

Sections 7-1,2 of the text. In particular, see Example 7-3.
Guide to Solving Work Problems.

Part A

Download and print this template. Then write your responses.

Part B

Download and print this template to record your data and calculations. This problem is a bit different than the usual problem in that you'll take the data that you need for the calculations. Set up to collect the data now. You'll use the same surface that you did for L122, the friction lab. Begin by propping one end of the surface up so that it's inclined at an angle of about 30^o. Make sure the plane is secure so that it won't slip. If it slips, that will change the angle.
1. Data: Record your data and calculations of angle and total weight in Table 1 of the template.
  1. Use a meter stick or tape to measure the hypotenuse and the rise for calculating the angle that the surface makes with the horizontal. (See Part B of L122 if you need to refresh your memory about what distances to measure.)
  2. Examine your data and results from L122. Transcribe the following values to this assignment: i) weight of the friction box, ii) coefficient of kinetic friction between the box and the surface. (If you determined the value of μ_k incorrectly, you'll need to correct that analysis first in order to obtain the correct value of μ_k to use in the present assignment.)
  3. Place the box on the surface, and place all three of the standard masses (total of 0.350 kg) in the box.
  4. Lay the spring scale on the surface with the hook headed down the surface. Zero the scale. Then with the 20 N spring scale held parallel to the plane, pull the box up the plane at constant velocity. Record the value of the tension force as T_u.
  5. Repeat part iv with this difference. Lay the spring scale on the surface with the hook pointed up the plane. Zero it again. Then pull the box down the plane under the same conditions as the previous part. Record the value of the tension force as T_d.
  6. Estimate the distance that you pulled the box along the plane. The particular amount isn't important, since it will be the same factor all your calculations of work. Record your estimate as d.
2. Pulling the box up the plane: Record the following in Table 2.
  1. For each force other than the net force, draw the F-d diagram and write the symbolic expression for calculating the work done by the force.
  2. For all but the work done by the net force, use your work expressions to calculate the values of the work. For the work done by the net force, enter the sum of all the other values of work.
3. Pulling the box down the plane: Complete Table 3 in a similar fashion to Table 2.
4. What value do you expect for the work done by the net force and why? Comment on the accuracy of your results.
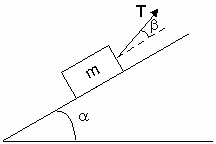A block of mass m is pulled from rest a distance d along an inclined plane by a force T applied through a rope that makes an angle β with the plane. The plane makes an angle α with the horizontal. The coefficient of kinetic friction between the block and the plane is μ_k.
1. Determine an expression for the work done by the force of kinetic friction. Give your expression in terms of m, d, μ_k, T, α, β, and g. Show your work completely. A net force problem is part of the solution. Be sure to keep the angles straight. There are 3 angles: α, β, and the angle θ between force and displacement.
2. Using the values below, calculate the value of the work done by the force of kinetic friction.
m = 2.00 kg
d = 1.80 m
μ_k= 0.350
T = 20.0 N
β = 15.0°
α = 30.0°