Rotation and Translation

Guide 10-2. Rotation and Translation

Related reading: Walker 10-4 (This reading doesn't provide as much detail as the information below.)

For most of our work so far, we've treated objects as idealized mass points. When describing rotational motion, we must treat objects as mass distributions. An object must have extent in space in order for it to make sense to speak of rotation of the object. We limit our studies of rotational motion to objects that are rigid. This means that the points of the object don't move relative to each other. This describes many solid objects. When a rigid object rotates, all points of the object have the same angular velocity.

A wheel rolls on a surface due to the static friction between the wheel and the surface. Without such friction, the wheel would slide without rolling. The latter motion, which is simply linear motion, is termed translation. In translation, all points of a rigid body move with the same linear velocity. If, on the other hand, the axle of the wheel is fixed in place and the wheel is then rotated, different parts of the wheel have translational velocities that are proportional to their perpendicular distances from the axis of rotation. In the case that the wheel rolls along a surface without sliding, each point of the wheel undergoes a combination of translational and rotational motion. We examine next the situations of i) pure rotational motion, ii) pure translational motion, and iii) rolling motion.

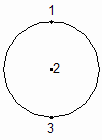
We'll examine the linear velocities of 3 points on a wheel or disc. These are shown in Figure 1. Point 1 designates the top of the wheel, Point 2 designates the center, and Point 3 the bottom. Note that Points 1 and 3 do not rotate with the wheel but rather always describe the top and bottom of the disc in the same sense that the center of the disc is always the center.

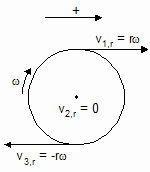
Figure 2 shows the disc in pure rotational motion. An example would be a car spinning its wheels while lifted off the ground on a rack. We take the direction of positive displacement to be to the right and the magnitude of the angular velocity of the disc to be ω. The linear velocities of the top, center, and bottom of the disc are then v_1,r = rω, v_2,r = 0, and v_3,r = -rω. Note that we are treating the symbol v as a vector and the symbols r and ω as magnitudes. Thus, for example, we explicitly use a negative sign to indicate velocites to the left. The subscript, r, on the velocities indicates pure rotation.

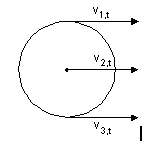
Figure 3 shows pure translational motion. This would describe, for example, the wheels of a car on the flat bed of an auto carrier traveling along the highway. It would also describe a disc sliding on a perfectly frictionless surface. (The latter may be difficult to imagine, since in any real-world situation, there is static friction which causes rotation.) In the case of pure translation, all points of the disc have the same velocity, therefore, v_1,t = v_2,t = v_3,t. Note that we use the subscript t to denote pure translation.

Figure 1	Figure 2	Figure 3	Figure 4	Figure 5

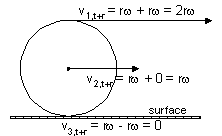Points on a wheel	Pure rotational motion	Pure translational motion	Rolling with slipping	Rolling without slipping

Now imagine that the disc moves along a horizontal surface. Static friction with the surface imparts rotational motion to the disc so that it has a combination of rotational and translational motion. This is the nature of rolling motion. Rolling can occur with or without slipping. Figure 4 above shows the more general situation with slipping. The linear velocity at each point is the vector sum of the translational and rotational components. Then the center of the disc, Point 2, has translational velocity only. We'll use a single symbol v_t = v_1,t = v_2,t = v_3,t to represent the translational velocity component at all points of the disc. With this notation, v_2,t+r = v_t. At the top of the disc, the translational and rotational velocity components add to give v_1,t+r = v_t + rω. At the bottom, the components subtract to give v_3,t+r = v_t - rω.

A Special Case and a Demonstration

Consider the idealized situation shown in Figure 5 above in which there is pure rolling (no slipping). This is the case we consider in many problems dealing with rolling motion, because that situation is simpler than if sliding must also be considered. When there is no slipping, the translational component of the velocity is v_t = rω. Since v_r = rω also, there is potential for confusion here. Hence, we make these important distinctions:

When a rigid body rotates with angular velocity, ω, the rotational velocity component of a point a perpendicular distance, r, from the axis of rotation is v_r = rω. This is the case whether the object is rolling or spinning in place.
When an object moves with a combination of rotation and translation, the translational velocity is the velocity of the center of mass of the object, that is, v_t = v_cm.
When an object rolls without slipping, the translational and rotational velocity components are equal. Thus v_t = v_r = rω. If there is slipping, v_t may be more or less than rω. An example in which v_t < rω is a disc rolling with slipping on a horizontal surface. An example in which v_t can be greather than rω is a disc rolling with sliding down an incline.

With the above in mind, we substitute v_t = rω to see the interesting fact that the velocity of the top of the disc is v_1,t+r = v_t + rω = rω + rω = 2rω, while the velocity at the bottom is v_3,t+r = v_t - rω = rω - rω = 0. The latter result appears non-intuitive to many people, because the disc as a whole is obviously moving. But recall that we're not treating Point 3 as a material point that moves with the disc. Point 3 is always the lower most point, and this point, as we have shown with the reasoning above, is instantaneoulsy at rest.

View this clip for a demonstration that provides visual evidence. (Download the clip to your hard drive and play it there rather than trying to play it in a web browser.)

Note that translational motion doesn't have to be straight-line motion.

thrown hammer Consider a hammer tossed in the air as shown in the photo to the left. The hammer rotates about the center of mass as the center of mass translates along a parabolic trajectory under the influence of gravity.