L159. Characteristics of a Light Bulb

About your report: Write your report on paper to scan later. Number the items of your report the same as numbered in the instructions.

 Goals

• to light a bulb
• to experimentally show that an incandescent light bulb does not obey Ohm's Law
• to determine the temperature of the bulb filament

 Equipment

Multimeter
Light bulb and holder
4 1.5-V batteries and battery holder(s)
Clip wires

 Prelab

1. Successfully complete the Multimeter Tutorial.
2. Read the introduction to this lab.
3. Read sections 21.2,3 of the text.
4. Watch this video to see what happens when a lit bulb is shot with a BB. (Don't try this with your bulbs at home!) When exposed to oxygen, the filament bursts into flame.

 Introduction

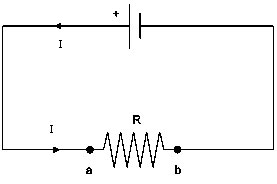The Definition of Resistance

The definition of electrical resistance is R = -ΔV_r/I, where ΔV_r is the potential difference across the resistor when conventional current I passes through the resistor. Consider the simple circuit with a battery and resistor shown to the right. Conventional current leaves the battery from the positive terminal and passes through the resistor from left to right. Point a has higher potential than point b, so the difference of potential across the resistor is ΔV_r = V_b - V_a < 0. It's conventional practice when measuring voltage with a meter to place the positive probe at the higher potential side. If we call V the voltage measured by a meter in this way, then V = V_a - V_b > 0. Therefore, V = -ΔV_r > 0. With this definition of voltage V as measured by a meter, the definition of resistance is R = V/I. As you can see, there's potential (no pun intended) to get confused by the meanings of V and -ΔV_r, besides the fact that V also represents the unit of the volt. We summarize these results in the table below.

Resistance of a Wire

The above is a treatment of the subject of electrical resistance in general. As a definition, R = -ΔV_r/I applies to any electrical resistor. In the specific case of a wire, the resistance can be determined from physical characteristics of the wire: length, cross-sectional area, type of material. The resistance of a wire is given by R = ρL/A, where ρ is the resistivity of the material, L is the length of the wire, and A is the cross-sectional area of the wire. A fact mentioned only briefly in the text is that the resistivity is temperature dependent. The resistivity at temperature T is given by ρ = ρ_o[1 + α(T - T_o)], where ρ_o is the resistivity at some reference temperature (typically 20 °C), and α is the temperature coefficient of resistivity of the material and is relatively constant. The units of α are °C^-1. For a wire of given length and cross-sectional area, the resistance will increase with temperature if α is positive. This fact is exploited in this experiment in order to determine the temperature of an incandescent bulb filament.

To Obey Ohm's Law or Not

R = -ΔVr/I and R = V/I are not statements of Ohm's Law. Instead, they are statements of the definition of resistance. Don't confuse them with Ohm's Law, which is discussed below.

Something to keep in mind as you read section 21.2 of the text is that the so-called Ohm's Law is not a physical law in same sense as Coulomb's Law or the law of gravitation. We think of a physical law as something that nature always obeys. Ohm's Law is really more a rule in the sense that many circuit elements don't obey it. In modern electronic circuits, in fact, Ohm's Law is probably violated more often than not. This is not to say that the relationship R = V/I isn't valid. You can always use that, because it's the definition of electrical resistance.

In order to determine whether a circuit element obeys Ohm's Law, one measures the current in the element as a function of the potential difference across the element. If a graph of current vs. potential difference is linear, then the circuit element obeys Ohm's Law. Here is the statement of Ohm's Law that you should remember:

A circuit element obeys Ohm's Law when the current in the element is proportional to the potential difference across the element. A graph of current vs. potential difference will be linear. In symbolic form, Ohm's Law is I = KΔVr, where K represents a constant. From the definition of resistance, we can see that the constant must be K = -1/R.

In order to determine in an experiment if a particular resistor obeys Ohm's Law, you would connect the resistor in a simple circuit to different numbers of batteries. For each number of batteries, you would measure the current in the circuit and the potential difference across the resistor. If you use the conventional method of placing the positive probe of the meter on the higher potential side of the resistor, then you would be measuring voltage, V. The voltage would be the independent variable and would be plotted on the horizontal axis in a graph of I vs. V. If the graph were linear, one would expect the slope of the linear fit to be 1/R. (Why wouldn't you expect the slope to be -1/R?)

Power Dissipation in Resistors and Incandescent Bulbs

The power provided by a battery is P_b = I|ΔV_b|, where ΔV_b is the potential difference across the battery terminals, and I is the current in the circuit. The power dissipated in a resistor is P_r = I²R. An alternative formula is P_r = (ΔV_r)²/R, where ΔV_r is the potential difference across the resistor. (See Guide 21-1b.) The same symbol I is used in both cases, because the current is the same in the battery and the resistor. In a given amount of time, Δt, the energy provided by the battery is U_b = P_bΔt, and the energy converted by the resistor to heat is U_r = P_rΔt. If we assume that the wires have negligible resistance and therefore negligible power dissipation, then for energy to be conserved, U_b - U_r = 0. Since the time interval is the same, P_b = P_r also.

An incandescent bulb is a resistor. The tungsten filament of the bulb is designed to get so hot that it gives off light when current passes through it. See the side-by-side photos to the right showing the filmament with current off and on. Due to the fact that the resistance of the filament is temperature-dependent, incandescent light bulbs do not obey Ohm's Law.

Incandescent light bulbs convert electrical energy to two other forms: thermal energy and radiant energy (light). Taking light to be the useful energy output of the bulb, the efficiency of an incandescent bulb is the ratio of radiant energy output to electrical energy input. For typical incandescent bulbs, this is very low, only a few percent. This low efficiency is a primary reason for the switch to fluorescent bulbs, which have efficiencies as much as 4 times greater.

 Part A. Lighting the bulb

The diagram to the right shows a bulb connected to a battery. The bulb is represented by a circle with a sawtooth line inside. This doesn't help you when you actually need to connect wires to a bare bulb to make it light. For this part, use a fresh battery, the bulb, a battery holder, and alligator leads. Do not use the light bulb holder, as that will defeat the purpose of this exercise. You may need another person to help you hold some of the parts. The goal is to light the bulb. This amounts to figuring out where on the bulb the electrical contacts are. Simply touch the alligator clips to those contacts wherever you think they are. If the bulb doesn't light but you feel the battery getting warm, you've created what's called a short circuit. That means you're connecting the battery to the same point on the bulb. This effectively bypasses the bulb and would quickly run down the battery if you left it connected that way. Once you're successful in lighting the bulb, sketch a large diagram showing the bulb and the two points on the bulb that you touched with the alligator clips. (If no matter what you do, you can't get the bulb to light, your battery may be too weak. In that case, try using two batteries in series.)
Having determined how to light a bulb, do so again, but reverse the positions of the alligator clips where they touch the bulb. The point is to make the current flow the opposite direction. Do you see a difference from the results of step 1? Did you expect to? Maybe you thought the above exercises were a bit too simple for high-school students. However, studies show that many college physics students don't know how to light a bulb when it's not in a socket. Screw the light bulb into its holder now. There are two clip contacts on the holder. Connect an alligator clip to each one. Touch the other ends of the alligator clips to the ends of a battery. If your battery is fresh, the bulb should light, although it will be weak. If the bulb doesn't light, make sure it's screwed completely into the holder. From now on you'll leave the bulb in its holder.
At this point, you should have your bulb in its holder connected to a single battery. Add a second battery in series with the first as shown to the right. Repeat with 3 batteries. Describe what you observed as you added batteries. What you're doing when you change the number of batteries is changing the amount of power dissipated by the bulb. Explain your observations using circuit concepts and relationships. The purpose of this question is to give you practice in using standard physics vocabulary such as potential difference, current, resistance, power and energy.  When you talk about potential difference, say potential difference across the bulb. Similarly, you would speak of current in the bulb. The goal is to explain clearly without making nonsensical or ambiguous statements about electrical circuits. Don't rely on equations to make your argument for you. Give your argument in paragraph form.

 Part B. Determining whether the bulb obeys Ohm's Law

Before making resistance, voltage, and current measurements, review the multimeter tutorial for how to connect the probes to the meter and to the circuit.

4. First, you'll measure what we call the room-temperature resistance of your light bulb, that is, the resistance when the bulb isn't lit. Ideally, you need to know the resistance of your bulb when no current is passing through it. In this case, the bulb will be at room temperature, which is typically 20 - 25 °C. However, when you connect your meter to the bulb to measure the resistance, the meter passes a small current through the bulb.This will heat the filament slightly and change its resistance. In order to minimize this effect, don't leave the meter connected for a long period of time while you measure the resistance. Just hold the leads in place long enough to get a stable reading. Record the reading as R_o.

5. Measure the voltage across the bulb for 1, 2, 3, and 4 batteries. (Will the voltage be positive or negative? If you don't know the answer, review the Introduction.) Record your results in a table like the following.

Room temperature resistance, Ro = _________ ohm
No. of batteries	Voltage V across bulb (V)	Current I in bulb (A)
1
2
3
4

6. Now measure the current the circuit for the same 4 combinations of batteries and record in the table. Remember to break the circuit and change the position of the red probe for measuring current.

7. Plot a graph of I vs. V in Logger Pro. Set both of the scales to start from the origin. (Which variable will go on the horizontal axis?) You won't turn in this LP file. Instead, include the following in your report: A half-page size, hand-drawn graph of I vs. V for the bulb. You don't have to show grid lines, but do draw the axes with a straightedge, number the scales, and place the 4 data points in approximately correct locations. The origin must show on your graph, as this is important to the interpretation in the next item.

8. We know that the current should be 0 when the voltage is 0. If the origin were in fact a data point, describe the overall trend of the data. Which way would the best-fit line passing through the origin and the data curve? How would the slope change with increasing potential difference? From this examination, describe how the resistance of the bulb changes with V and explain how you know this.

 Part C. Calculating the temperature of the filament

9. Using relationships from the introduction as starting points, show that for a wire of a given length and cross section, the wire's resistance is given by: R = R_o[1 + α(T - T_o)].

10. Look up the value of the temperature coefficient of resistivity for tungsten.

11. Calculate the highest temperature that the bulb filament reached. Start with a formula, solve for T, and show your substitutions. Round to appropriate significant figures.

 Conclusion

Summarize what you did and what you learned in this lab.

 Submitting your work

Scan and upload your report to BrainHoney. 

© North Carolina School of Science and Mathematics, All Rights Reserved. These materials may not be reproduced without permission of NCSSM.