This is a simulated lab that uses data obtained from an applet. |
Goals
- To determine an unknown charge using Coulomb's Law in a graphical analysis
- To apply the result to solve a related problem
Prelab
Complete and submit the following problem to BrainHoney L157PL.
 View this animation. The experiment shown in the animation can be
carried out in the laboratory in order to verify the inverse-squared
distance form of Coulomb's Law. However, it's a difficult experiment to
do well and achieve good results. That's because in the real world,
charge leaks off of objects due to water vapor in the atmosphere. You'll do the theory for the experiment by developing an equation between the relevant variables. Refer to the
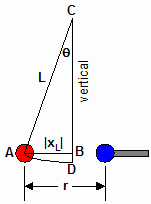
diagram to the right for definitions of symbols.
View this animation. The experiment shown in the animation can be
carried out in the laboratory in order to verify the inverse-squared
distance form of Coulomb's Law. However, it's a difficult experiment to
do well and achieve good results. That's because in the real world,
charge leaks off of objects due to water vapor in the atmosphere. You'll do the theory for the experiment by developing an equation between the relevant variables. Refer to the
diagram to the right for definitions of symbols.
L = length of the string from point of support C to the center of the red charged sphere at A
θ = angle that the string makes with the vertical, ACD
|xL| = AB is the absolute value of the horizontal deflection of the left ball from the vertical
r = separation between the centers of the two charged spheres
m = mass of either sphere
Q = charge on either sphere
k = electrostatic constant
Begin with a diagram of the forces acting on the red charge for the situation when the charge is in equilibrium from the electrical, weight, and tension forces acting on it. Then carry out a net force analysis to determine the relationship between |xL| and r. Expect to obtain an equation in the form |xL| = Af(r), where f(r) is a function of the separation r and A is a collection of constants that depend on k, L, Q, m, and g. See the notes in the yellow box below for help in dealing with the angle.
In order to eliminate θ from the equation, you'll need to use some approximations.
From the diagram, note that |xL| = Lsinθ. For sufficiently small angles sinθ is approximately equal to θ (in radians). Thus, |xL| is approximately equal to Lθ.
The arc length AD = Lθ. But AD is very nearly equal to AB which equals |xL|.
Combining results from 1 and 2, you'll be able to eliminate θ from your equation for |xL|.
Data and Analysis
In prelab, you derived the theory of the situation that you will investigate experimentally in this virtual lab. You'll take data from this animation. Recall that the result of the prelab was that |xL| = Af(r), where f(r) = 1/r2 and |xL| represented the horizontal distance of the left charge from its initial position at x = 0. Another way of saying this is that |xL| represents the deflection of the left charge caused by the right charge. Therefore, |xL| will be the dependent variable in the investigation to follow.
The independent variable is the separation, r, of the two charges. When you take data from the applet, you'll have two position outputs, xL and xR, the positions of the left and right charges respectively. You'll calculate r using those two positions. (Caution: Don't simply add the positions, since one of them is negative. You need the total distance, between the charges.) You'll then plot a graph of |xL| vs. r, re-express r appropriately to obtain a linear fit, and use the slope of the fit to determine the value of the unknown charge.
-
Collect data from the applet for the positions of the left and right charges. Record these in two columns of the data table. Then create columns to calculate the corresponding values of r and 1/r2. Plot and fit |xL| vs. 1/r2.
-
Construct the matching table and write the equation of fit in a textbox.
Do the following on a second page of your LP file.
-
Tell how one knows that both spheres have the same charge after they are touched together. The phrase same charge means not only the same sign but also an equal amount of charge. For a complete explanation, you'll need to use all of the following: i) the physics of charge transfer, ii) the law of conservation of charge, iii) the fact that the spheres have identical size, shape, and composition. Make it clear in your response how you use each of the three things.
-
Use the slope of your fit together with the result of the prelab to determine the unknown charge Q. Show that the units reduce correctly.
- Is the intercept of your fit the value that you expect? Explain. You'll need to describe what it means for f(r) to approach 0 and how this affects the left sphere. (Saying that a deflection of 0 is expected when the separation is 0 should be obviously incorrect to you.)
Application
Do the following on a third page of your LP file.
Here's a related situation. Open this animation, read the problem description, and run the applet. You should see how you can apply the equation you already have from the prelab to this new solution. With that in mind, do the following.
-
Use the same symbols as for the prelab problem. However, you should be able to see how to eliminate the symbol, |xL| by expressing it in terms of the separation r. Do that and then solve for r in terms of k, L, Q, m, and g.
-
Using the value of Q from step 4 of Method and Analysis above and the constants given in the applet, calculate the separation of the spheres.
-
Suppose you have a third sphere, identical in size and composition to the other two but neutral in charge. The sphere is attached to an insulating handle that you hold. You touch the third sphere to the left one and then quickly withdraw. The left sphere swings down and touches the right sphere momentarily. Then the left and right spheres re-establish equilibrium. For the new equilibrium state, do the following.
-
Determine the values of charge on the hanging spheres. Explain why they have those values.
- Calculate the final equilibrium separation of the spheres.
-
Submitting your file
Submit your LP file to the L157 item in WebAssign.