Goals

1. To review and apply what you've learned about standing waves to waves on a string
2. To apply a method of analysis that will be used in a future lab
3. To investigate quantitatively the affect of tension on waves on a string

 Introduction

For this experiment, two relationships involving wave speed are needed. One of them comes from the formula v = Δd/Δt and is λ = v/f.  The other relates to the elasticity and inertia of the medium. This relationship is:

Wave speed = (Tension/Linear density)^1/2

Linear density is the ratio of the mass of a unit length of the string to that length.  In terms of the symbols F_T for tension and μ for linear density,

v = (F_T/μ)^1/2.

It's important to realize that the latter relationship is what determines the speed of waves on a string.  The relationship λ = v/f doesn't determine the speed of waves on a string; λ = v/f simply describes the motion without addressing the causes of the motion. An analogous situation has to do with the relationships a = F_net/m and a = Δv/Δt. Newton's 2nd law says the cause of the acceleration of an object is the sum of the forces on the object, while a = Δv/Δt describes how the velocity changes with time without saying why it changes.

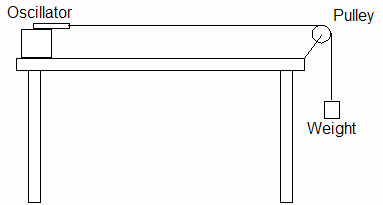
The apparatus used in this demonstration produces standing waves on a horizontal string. See the diagram to the right. There are nodes at each end of the spring. One end is attached to a driver that oscillates at a constant frequency. The other end passes over the end of a pulley and has weight hanging on it to produce tension. By adjusting the frequency of the oscillator or the tension in the string, standing waves with various numbers of antinodes can be produced.

 Prelab

1. Read the guide, Wave Speed and What It Depends On.
2. View the video clip, Standing Waves on a String: Streamed / RealPlayer / Flash. As you watch the video, record the 4 frequencies as they're displayed on the meter. You'll need the values for one of the L148PL questions.
3. Complete L148PL.

 Experimental Design

We'll discuss answers to the following in the WebEx session. All students will be expected to participate in providing responses.

1. Describe a method to accurately determine the linear density of the string. (Hint: Simply consider how linear density is defined. Then think of how to get an accurate measurement.)

2. How can you calculate the tension in the string? What assumption(s) is(are) involved?

3. Given the weight hanging from the string and the linear density, how can you calculate the wave speed in the string?

4. Draw the standing wave pattern of the fundamental frequency.

5. If the length of the string is L, what is the wavelength of the fundamental?

6. What frequency do we need to set for the oscillator to generate the fundamental?

7. What if we wanted to generate the 2nd harmonic? What frequency would be needed?

8. Will this change in the frequency change the wave speed? Explain.

9. How could we change the wave speed to make it, say, 25% greater than its present value?

10. If we changed the wave speed, how would the wavelength have to change to produce 2 antinodes?

11. For the greater wave speed, how would the frequency have to change to produce 2 antinodes?

12. Suppose one did a linear fit to a plot of f_n vs. n for the n = 1 to 3 data. How would one use the slope of the fit to determine the wave speed? What additional information would be needed?

13. Why wouldn't the n = 4 data from the video clip be included in the analysis of step 12?

 Analysis & Interpretation

1. Carry out the analysis as discussed in item 12 of the Experimental Design. Include the usual matching table and equation of fit in a textbox. (There will not be an expected value for the slope.) Upload your file to WA L148.

Continue with WA L148 in answering the following.

2. The length of the vibrating string wasn't recorded. For calculations to follow, you'll need a value for the length of the vibrating string. Estimate the length to 2 significant figures. Consider the fact that a person's arm span is approximately equal to their height, and the instructor is 5'11" tall.

   1. Use the slope of your fit of f_n vs. n to calculate the speed of waves on the string.

   2. Calculate the linear density of the string.

   3. Calculate the total mass hanging from the string after the instructor added more mass.

   4. Is your answer for the last part reasonable? Explain.

 Conclusion

You need not write a conclusion for this lab exercise.

© North Carolina School of Science and Mathematics, All Rights Reserved. These materials may not be reproduced without permission of NCSSM.